Cell colonies and branching patterns
Benoît PERTHAME
Lab. J.-L. Lions, UPMC Paris 6
There are very few PDE models undergoing branching instability. Among those, one of the most famous, inspired by dentritic growth of cell populations is known as Mimura's model. It describes the growth of a cell population under the effect of a nutrient which is locally depleted.
We present a conservative parabolic model that undergoes branching instabilities. The swarmers are modeled by a Fokker-Planck type equation à la Keller-Segel, coupled with two fields describing attraction and repulsion. It also includes the 'quorum sensing' limitation proposed by Dolak and Schmeiser.
Several reduced models explain stability and unstability of plateau type traveling wave solutions.
This lecture is based on collaborations with F. Cerreti, Ch. Schmeiser, M. Tang and N. Vauchelet.
IMAGES
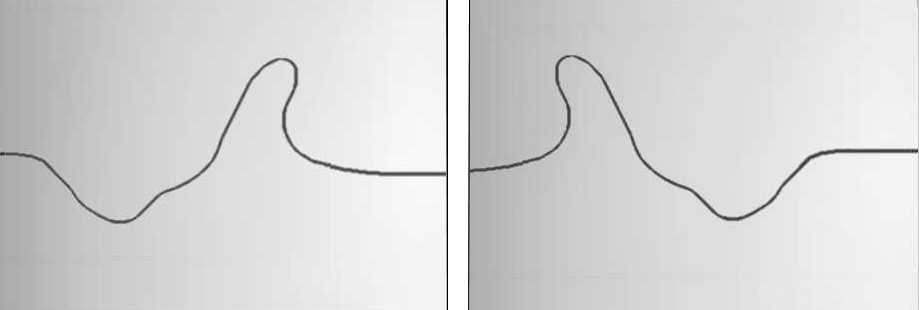
Onde solitaire stationnaire de symétrie impaire (plus de détails...)
CONFÉRENCES
Auto-organisation en physique et en biologie, morphogenèse, ondes-particules, turbulence, physique non linéaire, Colloque International en mémoire d’Yves Couder à l'ENS et UPCité, 4 Juin 2024
Bifurcations and Instabilities in Fluid Dynamics, Edinburgh, Scotland, 24 Juin 2024
1st European Fluid Dynamics Conference - EFDC 2024, Aachen, Germany, 16 Septembre 2024